

Sequences have many real-world applications in various fields, including science, finance, and technology. Periodic sequences can exhibit some interesting behaviors, such as the existence of sub-sequences with different properties. The sum of the first n terms of an arithmetic sequence can be calculated using the formula:Īrithmetic sequences are used in many real-world situations, such as calculating the depreciation of assets over time or the regular payment of loans.Įxample: diverges as n approaches infinity.Ī sequence is said to be periodic if its terms repeat after a certain number of terms, as described above. Where a_n is the nth term, a_1 is the first term, n is the index of the term, and d is the common difference. The formula for an arithmetic sequence is: Arithmetic SequenceĪn arithmetic sequence is a type of sequence where each term is obtained by adding a constant value, called the common difference, to the previous term. A sequence can be defined either explicitly, by giving a formula for each term, or recursively, by giving a rule for computing each term based on the previous terms. The index of a term refers to its position in the sequence, starting from the first term. The terms of a sequence are the values that the function outputs for each natural number input. Definition and TermsĪ sequence is a function that maps the natural numbers to a set of numbers. In this article, we will explore the different types of sequences, their properties, and their applications.
/7-downdog-56f98e3d3df78c7841935724.jpg)
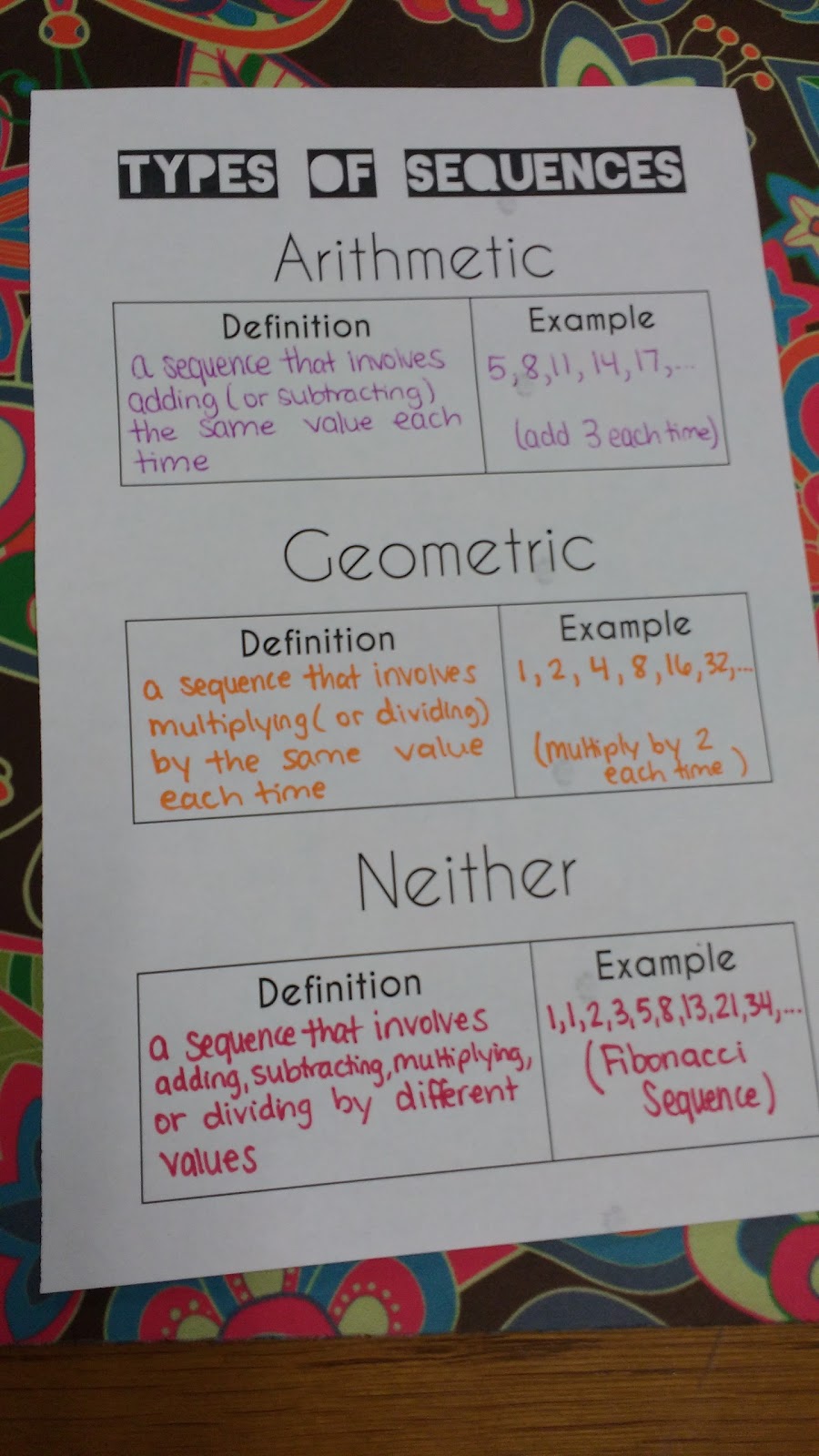
They are used in many different areas of mathematics and have numerous real-world applications. Sequences are a fundamental concept in mathematics that involve ordered lists of numbers that follow a pattern or rule. Types of Sequences: Definition, Examples, and Properties


 0 kommentar(er)
0 kommentar(er)
